A simple solution would be to run a linear search on the array and find the largest integer in the array less than or equal to x and the smallest integer in the array greater than or equal to x.
The floor and the ceiling of an integer.
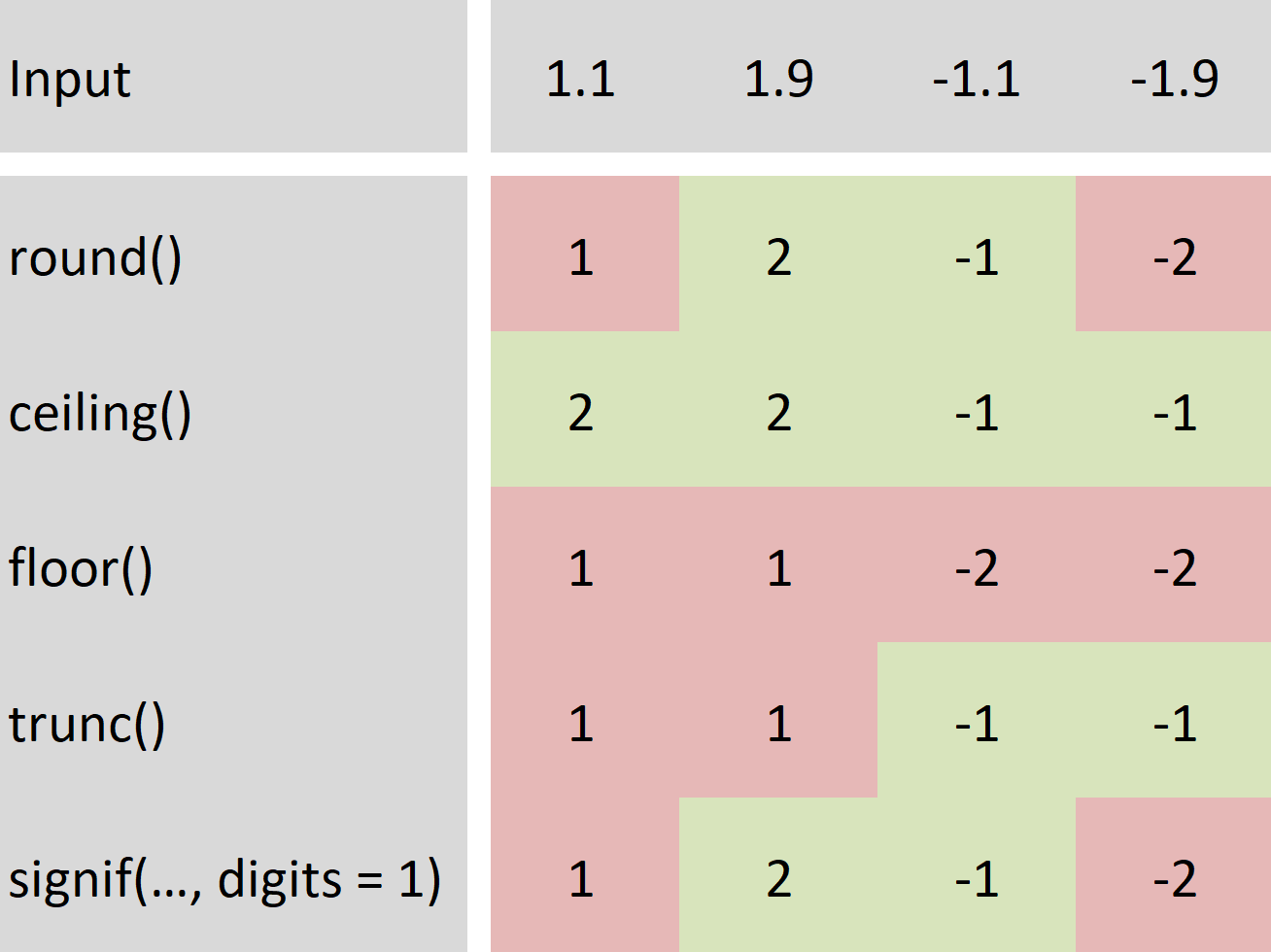
The reason for this is historical as the first machines used ones complement and truncation was simpler to implement floor is simpler in two s complement.
That would be the floor and ceiling of number x respectively.
Some say int 3 65 4 the same as the floor function.
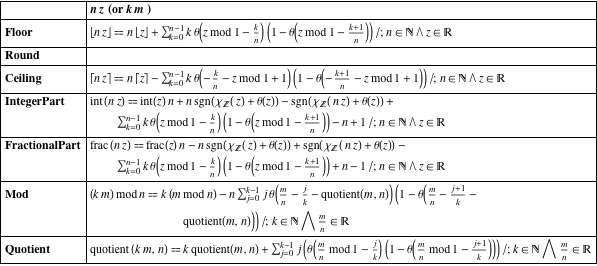
Floor and ceiling functions problem solving problems involving the floor function of x x x are often simplified by writing x n r x n r x n r where n x n lfloor x rfloor n x is an integer and r x r x r x satisfies 0 r 1.
In mathematics and computer science the floor and ceiling functions map a real number to the greatest preceding or the least succeeding integer respectively.
Rounds downs the nearest integer.
0 r 1.
Returns the largest integer that is smaller than or equal to x i e.
2 2 4 x 2 d x.
Here x is the floating point value.
As with floor functions the best strategy with integrals or sums involving the ceiling function is to break up the interval of integration or summation into pieces on which the ceiling function is constant.
And this is the ceiling function.
Number 10 ceiling is 1 floor is 9.
In most programming languages the simplest method to convert a floating point number to an integer does not do floor or ceiling but truncation.